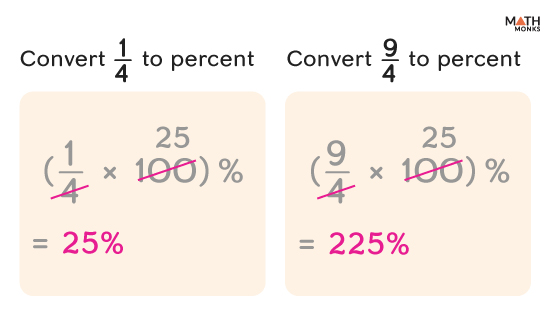To convert a fraction to a percent, we first convert it to decimal, multiply it by 100 to get the percentage value, and finally add a ‘%’ sign.
It helps us to compare and order two quantities more easily.
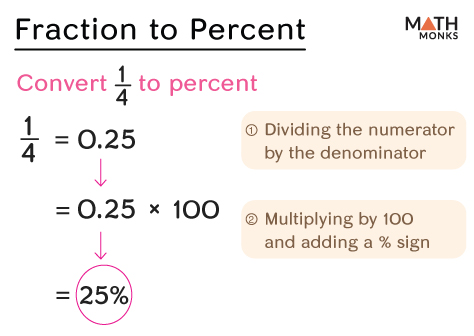
Let us convert ${\dfrac{1}{4}}$ to percent.
Dividing the Numerator by the Denominator of the Fraction
${\dfrac{1}{4}}$ = 0.25
Multiplying the Result by 100 and Moving the Decimal Point to the Right
0.25 × 100 = 25%
Here, the answer is less than 100% as ${\dfrac{1}{4}}$ is a proper fraction.
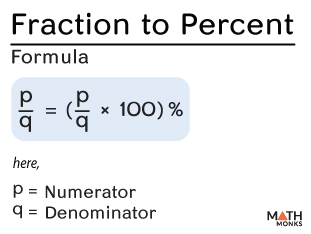
Formula
Thus, the formula to convert fraction ${\dfrac{p}{q}}$ to percentage is:
${\dfrac{p}{q}}$ = ${\left( \dfrac{p}{q}\times 100\right)}$ %
Here,
p = Numerator
q = Denominator
This is another way of converting a fraction to a percent where we multiply the fraction by 100, simplify it, and then add a ‘%’ sign.
For example,
${\dfrac{1}{4}}$ = ${\dfrac{1}{4}\times 100}$ = 25%
Alternative Method
In this method, we find the equivalent fraction with a denominator of 100. Then, the numerator with the ‘%’ sign represents the percent.
Let us convert ${\dfrac{1}{4}}$ into percent.
Multiplying the Denominator by a Number to get 100
Since 4 × 25 = 100
Thus, we multiply 25 by the denominator 4 to get 100
Multiplying the Numerator by the Same Number
${\dfrac{1\times 25}{4\times 25}}$ = ${\dfrac{25}{100}}$
Writing the Numerator with the ‘%’ Sign
Thus, the percentage is 25%
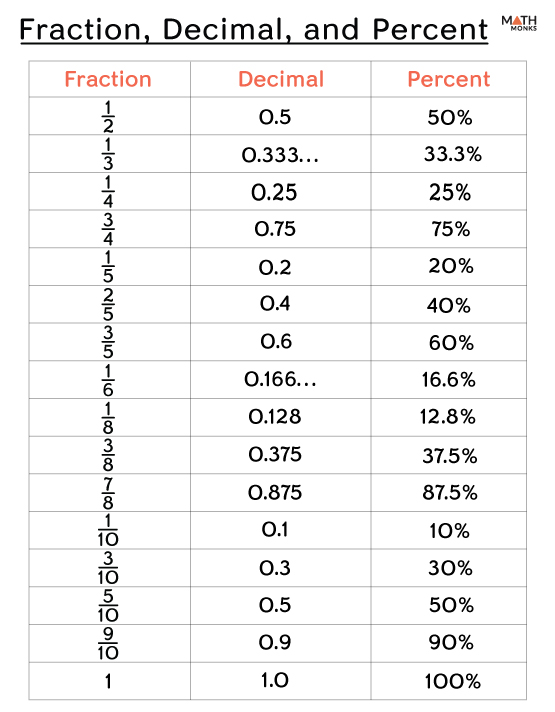
Fraction to Percent Table
Here is a fractions-to-percent conversion table for your use.
For Improper Fractions
While converting an improper fraction to a percent, we follow the same steps.
Let us convert ${\dfrac{9}{4}}$ to percent.
Converting to Decimal Number
${\dfrac{9}{4}}$ = 2.25
Multiplying the Decimal Number by 100
2.25 × 100 = 225%
Here, we observe that the answer is above 100%, as ${\dfrac{9}{4}}$ is an improper fraction.
Solved Examples
![]() What is ${\dfrac{13}{20}}$ in percent form?
What is ${\dfrac{13}{20}}$ in percent form?
Solution:
![]()
Here, ${\dfrac{13}{20}\times 100}$ = 65%
![]() What is ${\dfrac{2}{5}}$ as a percent?
What is ${\dfrac{2}{5}}$ as a percent?
Solution:
![]()
Here, ${\dfrac{2}{5}\times 100}$ = 40%
![]() What is ${\dfrac{12}{20}}$ as a percent?
What is ${\dfrac{12}{20}}$ as a percent?
Solution:
![]()
Here, ${\dfrac{12}{20}\times 100}$ = 60%
![]() Compare the fractions ${\dfrac{3}{4}}$ and ${\dfrac{16}{20}}$ by converting them to percentages.
Compare the fractions ${\dfrac{3}{4}}$ and ${\dfrac{16}{20}}$ by converting them to percentages.
Solution:
![]()
Here, ${\dfrac{3}{4}\times 100}$ = 75%
${\dfrac{16}{20}\times 100}$ = 80%
Since 80% > 75%
Thus, ${\dfrac{16}{20}}$ > ${\dfrac{3}{4}}$