To convert a percent to a fraction, we remove the ‘%’ sign, divide by 100, and then simplify the resulting fraction.
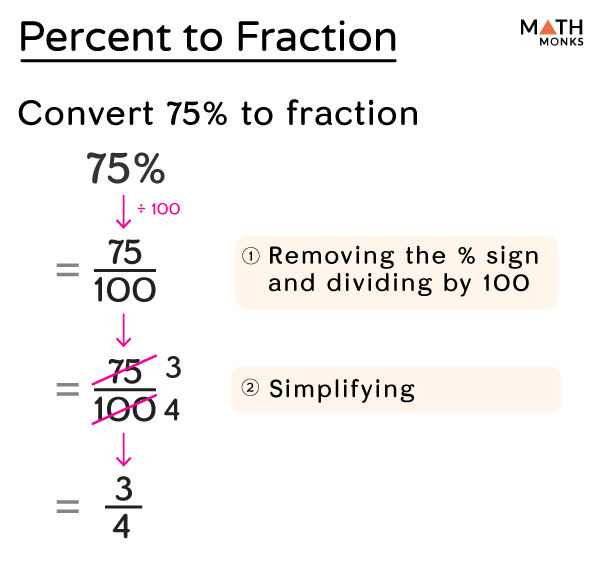
Let us convert 75% to a fraction.
Removing the ‘%’ Sign and Dividing by 100
75% = ${\dfrac{75}{100}}$
Simplifying
${\dfrac{75\div 25}{100\div 25}}$ = ${\dfrac{3}{4}}$
Thus, 75% = ${\dfrac{3}{4}}$
Here are some more percent-to-fraction conversions.
20% = ${\dfrac{20}{100}}$ = ${\dfrac{20\div 20}{100\div 20}}$ = ${\dfrac{1}{5}}$
40% = ${\dfrac{40}{100}}$ = ${\dfrac{40\div 20}{100\div 20}}$ = ${\dfrac{2}{5}}$
60% = ${\dfrac{60}{100}}$ = ${\dfrac{60\div 20}{100\div 20}}$ = ${\dfrac{3}{5}}$
80% = ${\dfrac{80}{100}}$ = ${\dfrac{80\div 20}{100\div 20}}$ = ${\dfrac{4}{5}}$
For Mixed Numbers
To convert mixed numbers, we first convert them to improper fractions, remove the % sign, and divide by 100.
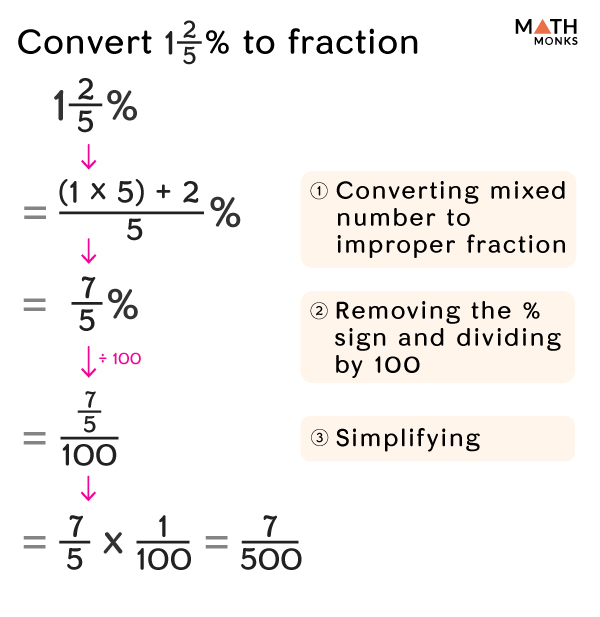
Let us now convert ${1\dfrac{2}{5}}$ %
Converting the Mixed Number to an Improper Fraction
${1\dfrac{2}{5}}$ % = ${\dfrac{\left( 1\times 5\right) +2}{5}}$ % = ${\dfrac{7}{5}}$ %
Removing the ‘%’ Sign and Dividing by 100
${\dfrac{\dfrac{7}{5}}{100}}$
= ${\dfrac{7}{5}\times \dfrac{1}{100}}$
= ${\dfrac{7}{500}}$, which can not be simplified or reduced further.
Thus, ${1\dfrac{2}{5}}$ % = ${\dfrac{7}{500}}
For Decimal Numbers
To convert any decimal number, we first remove the %sign, divide by 100, and then multiply both numerator and denominator by 10.
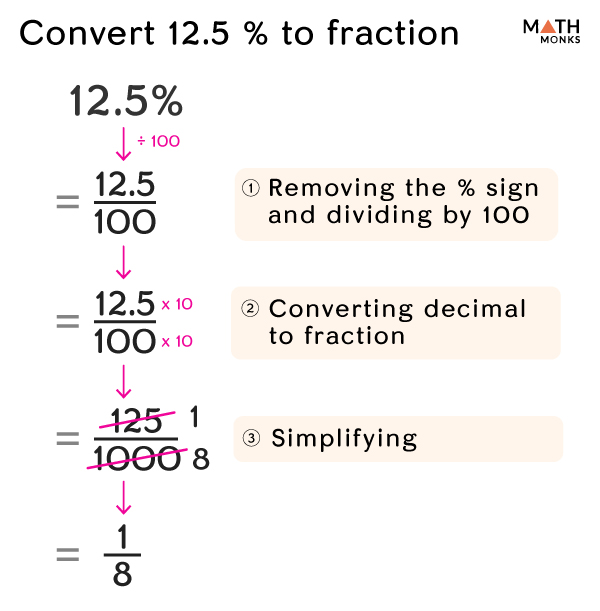
Let us now convert 12.5% to fractional form.
Removing the ‘%’ Sign and Dividing by 100
${\dfrac{12\cdot 5}{100}}$
Multiplying the Numerator and Denominator by 10 or Powers of 10
Here, we multiply both the numerator and the denominator by 10 for each digit after the decimal point.
Since there is 1 decimal digit in 12.5
On multiplying the numerator and the denominator by 10,
${\dfrac{12\cdot 5\times 10}{100\times 10}}$
= ${\dfrac{125}{1000}}$
= ${\dfrac{125\div 125}{1000\div 125}}$
= ${\dfrac{1}{8}}$
Conversion Table
Here is a percent-to-fraction conversion table for your use.
| Percent | Fraction |
|---|
| 1% | ${\dfrac{1}{100}}$ |
| 2% | ${\dfrac{1}{50}}$ |
| 4% | ${\dfrac{1}{25}}$ |
| 5% | ${\dfrac{1}{20}}$ |
| 10% | ${\dfrac{1}{10}}$ |
| 12% | ${\dfrac{3}{25}}$ |
| 12.5% | ${\dfrac{1}{8}}$ |
| 20% | ${\dfrac{1}{5}}$ |
| 25% | ${\dfrac{1}{4}}$ |
| 30% | ${\dfrac{3}{10}}$ |
| 40% | ${\dfrac{2}{5}}$ |
| 50% | ${\dfrac{1}{2}}$ |
| 60% | ${\dfrac{3}{5}}$ |
| 70% | ${\dfrac{7}{10}}$ |
| 75% | ${\dfrac{3}{4}}$ |
| 80% | ${\dfrac{4}{5}}$ |
| 90% | ${\dfrac{9}{10}}$ |
| 100% | ${1}$ |
Solved Examples
![]() Convert ${12\dfrac{1}{2}}$ % to fraction.
Convert ${12\dfrac{1}{2}}$ % to fraction.
Solution:
![]()
${12\dfrac{1}{2}}$ %
= ${\dfrac{\left( 12\times 2\right) +1}{2}}$ %
= ${\dfrac{25}{2}}$ %
= ${\dfrac{\dfrac{25}{2}}{100}}$
= ${\dfrac{25}{2}\times \dfrac{1}{100}}$
= ${\dfrac{1}{8}}$
Thus, ${12\dfrac{1}{2}}$ % = ${\dfrac{1}{8}}$
![]() Convert 68% to fraction.
Convert 68% to fraction.
Solution:
![]()
Here, 68% = ${\dfrac{68}{100}}$ = ${\dfrac{68\div 4}{100\div 4}}$ = ${\dfrac{17}{25}}$