Exponents and Square Roots

Exponents and square roots are inversely related operations. While exponents involve raising a number to a power, square roots inverse this operation by finding the original base that, when squared, gives the result.

For example, 

8 to the 2nd power is 8² = 64, which means 8 is multiplied by itself 2 times. When exponents are used, the results grow very rapidly. 

Now, to find the square root of 64, we need a number that, when squared (multiplied by itself), equals 64. That number is 8, so the square root of 64 is 8. 

Exponents to Square Roots

When converting an exponent into a square root, the base, say x, is raised to the fractional exponent, ${x^{\dfrac{1}{2}}}$, which represents the square root of that base. It can be expressed as 

${x^{\dfrac{1}{2}}=\sqrt{x}}$

In general, raising a base to the power of ${\dfrac{1}{n}}$ corresponds to finding the n^th root of the base.

For example,

${9^{\dfrac{1}{2}}=\sqrt{9}=3}$

Square Roots to Exponents

To express a square root as an exponent, the base under the radical is rewritten with a fractional exponent. It is expressed as 

${\sqrt{x}=x^{\dfrac{1}{2}}}$

More generally, an n^th root is written using a fractional exponent: ${\sqrt[n] {x}=x^{\dfrac{1}{n}}}$

For example,

${\sqrt{9}=9^{\dfrac{1}{2}}}$

However, to simplify complex problems involving these conversions, we need to follow certain rules.

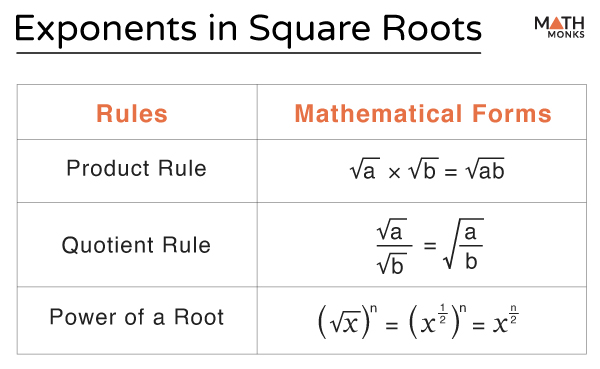
Rules

Here are the rules for simplifying expressions involving both square roots and exponents.

Solved Examples