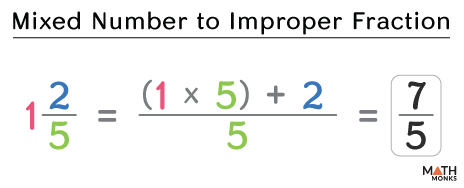
To convert a mixed number to its corresponding improper fraction, we follow the following steps:
Steps
Step 1: Multiplying the Denominator with the Whole Number
Here,
Denominator = 5
Whole number = 1
Now, by multiplying 5 and 1, we get
5 × 1 = 5
Step 2: Adding the Numerator to the Product
Here,
Product = 5
Numerator = 2
Now, adding 2 to it, we get
5 + 2 = 7
Step 3: Writing in Improper Fraction Form
Mathematically, the improper fraction is written as
${\dfrac{\left( Denominator\times Whole \ Number\right) +Numerator}{Denominator}}$
Thus, the improper fraction is ${\dfrac{7}{5}}$
Let us convert ${3\dfrac{1}{2}}$ into improper fraction,
Step 1: Multiplying the Denominator with the Whole Number
Here,
Denominator = 2
Whole number = 3
Now, by multiplying 2 and 3, we get
2 × 3 = 6
Step 2: Adding the Numerator to the Product
Here,
Product = 6
Numerator = 1
Now, adding 1 to it, we get
6 + 1 = 7
Step 3: Writing in Improper Fraction Form
${3\dfrac{1}{2}= ${\dfrac{7}{2}}$
Thus, the mixed number ${3\dfrac{1}{2}}$ as an improper fraction is ${\dfrac{7}{2}}$
Solved Examples
![]() Convert ${2\dfrac{2}{3}}$ to improper fraction.
Convert ${2\dfrac{2}{3}}$ to improper fraction.
Solution:
![]()
As we know, ${\dfrac{\left( Denominator\times Whole \ Number\right) +Numerator}{Denominator}}$
Here,
The denominator = 3
The numerator = 2
The whole number = 2
Now, ${2\dfrac{2}{3}=\dfrac{\left( 2\times 3\right) +2}{3}}$ = ${\dfrac{8}{3}}$
Thus, the improper fraction is ${\dfrac{8}{3}}$
![]() Write the mixed number ${7\dfrac{4}{13}}$ as improper fraction.
Write the mixed number ${7\dfrac{4}{13}}$ as improper fraction.
Solution:
![]()
As we know, ${\dfrac{\left( Denominator\times Whole \ Number\right) +Numerator}{Denominator}}$
Here,
The denominator = 13
The numerator = 4
The whole number = 7
Now, ${7\dfrac{4}{13}=\dfrac{\left( 7\times 13\right) +4}{13}}$ = ${\dfrac{95}{13}}$
Thus, the improper fraction is ${\dfrac{95}{13}}$
![]() Turn the mixed number ${6\dfrac{1}{6}}$ into improper fraction.
Turn the mixed number ${6\dfrac{1}{6}}$ into improper fraction.
Solution:
![]()
As we know, ${\dfrac{\left( Denominator\times Whole \ Number\right) +Numerator}{Denominator}}$
Here,
The denominator = 6
The numerator = 1
The whole number = 6
Now, ${6\dfrac{1}{6}=\dfrac{\left( 6\times 6\right) +1}{6}}$ = ${\dfrac{37}{6}}$
Thus, the improper fraction is ${\dfrac{37}{6}}$
![]() Change ${4\dfrac{1}{2}}$ into improper fraction.
Change ${4\dfrac{1}{2}}$ into improper fraction.
Solution:
![]()
As we know, ${\dfrac{\left( Denominator\times Whole \ Number\right) +Numerator}{Denominator}}$
Here,
The denominator = 2
The numerator = 4
The whole number = 1
Now, ${4\dfrac{1}{2}=\dfrac{\left( 4\times 2\right) +1}{2}}$ = ${\dfrac{9}{2}}$
Thus, the improper fraction is ${\dfrac{9}{2}}$