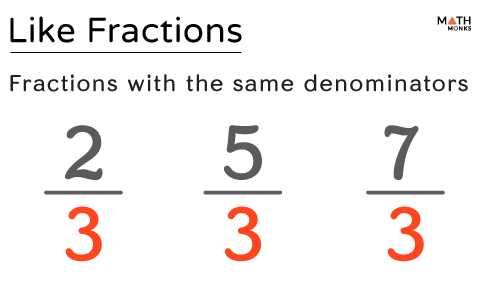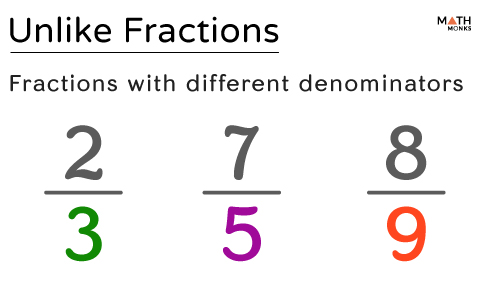Fractions are classified into different types based on their numerators and denominators.
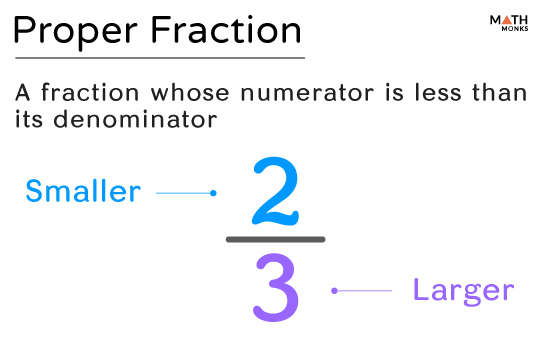
Proper Fraction
A fraction in which the numerator is less than its denominator is called a proper fraction. Its value is always less than 1.
${\dfrac{2}{3}}$, ${\dfrac{4}{5}}$, and ${\dfrac{2}{7}}$ are a few examples of proper fractions.
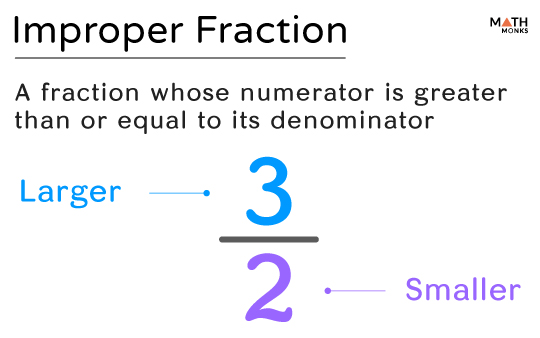
Improper Fraction
An improper fraction is a fraction in which the numerator is greater than or equal to its denominator. The value of a proper fraction is always greater than or equal to 1.
${\dfrac{3}{2}}$, ${\dfrac{5}{4}}$, ${\dfrac{7}{2}}$, and ${\dfrac{9}{9}}$ are a few examples of improper fractions.
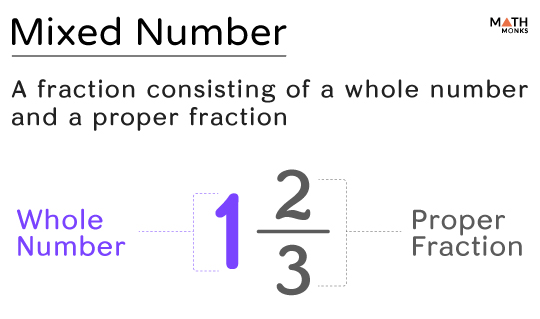
Mixed Fraction
A mixed fraction consists of a whole number and a proper fraction. Thus, they are always greater than 1.
${1\dfrac{2}{3}}$ and ${2\dfrac{3}{5}}$ are some examples of mixed fractions.
Like Fractions
Fractions with the same denominators are called like fractions.
${\dfrac{2}{3}}$, ${\dfrac{5}{3}}$, ${\dfrac{7}{3}}$, and ${\dfrac{8}{3}}$ are a few like fractions.
Simplifying such fractions is easy, as the denominators of all the fractions are the same.
For example,
${\dfrac{2}{3}+\dfrac{5}{3}-\dfrac{7}{3}+\dfrac{8}{3}}$
= ${\dfrac{2+5-7+8}{3}}$
= ${\dfrac{8}{3}}$
Unlike Fractions
Fractions with different denominators are known as unlike fractions.
${\dfrac{2}{3}}$, ${\dfrac{7}{5}}$, and ${\dfrac{7}{9}}$ are a few unlike fractions.
Simplifying such fractions involves factorizing the denominators before carrying out addition and subtraction.
For example,
${\dfrac{2}{3}+\dfrac{7}{5}}$
= ${\dfrac{2\times 5+7\times 3}{3\times 5}}$
= ${\dfrac{31}{15}}$
While multiplying, we first multiply the numerators and then the denominators.
For example,
${\dfrac{2}{3}\times \dfrac{7}{5}}$
= ${\dfrac{2\times 7}{3\times 5}}$
= ${\dfrac{14}{15}}$
In division, we multiply the dividend by the reciprocal of the divisor.
${\dfrac{2}{3}\div \dfrac{7}{5}}$
= ${\dfrac{2}{3}\times \dfrac{5}{7}}$
= ${\dfrac{2\times 5}{3\times 7}}$
= ${\dfrac{10}{21}}$
Equivalent Fractions
Equivalent fractions represent the same value after being simplified or reduced. To get equivalent fractions, we can multiply or divide the numerator and denominator of the given fraction by the same number.
For example,
${\dfrac{2}{3}}$ and ${\dfrac{6}{9}}$ are equivalent fractions.
Unit Fractions
Unit fractions are the fractions whose numerators are 1.
For example,
${\dfrac{1}{3}}$ and ${\dfrac{1}{9}}$ are unit fractions.
Here, we summarize the different types of fractions.
Solved Examples
![]() From the following fractions, identify the types of fractions as proper, improper, or mixed:
From the following fractions, identify the types of fractions as proper, improper, or mixed:
${\dfrac{1}{15}}$, ${1\dfrac{3}{8}}$, ${\dfrac{9}{3}}$, ${\dfrac{1}{7}}$, ${\dfrac{5}{5}}$, ${\dfrac{7}{3}}$
Solution:
![]()
Here,
Proper fractions: ${\dfrac{1}{15}}$, ${\dfrac{1}{7}}$
Improper fractions: ${\dfrac{9}{3}}$, ${\dfrac{5}{5}}$, ${\dfrac{7}{3}}$
Mixed fraction: ${1\dfrac{3}{8}}$
![]() Identify the like fractions:
Identify the like fractions:
${\dfrac{1}{5}}$, ${1\dfrac{3}{5}}$, ${\dfrac{9}{2}}$, ${\dfrac{2}{7}}$, ${\dfrac{3}{2}}$, ${\dfrac{10}{7}}$, ${\dfrac{10}{5}}$
Solution:
![]()
Here,
${\dfrac{1}{5}}$, ${1\dfrac{3}{5}}$, and ${\dfrac{10}{5}}$ are like fractions.
${\dfrac{9}{2}}$ and ${\dfrac{3}{2}}$ are like fractions.
${\dfrac{2}{7}}$ and ${\dfrac{10}{7}}$ are like fractions.
![]() Write two equivalent fractions of ${\dfrac{12}{15}}$
Write two equivalent fractions of ${\dfrac{12}{15}}$
Solution:
![]()
Here, ${\dfrac{12\div 3}{15\div 3}=\dfrac{4}{5}}$
${\dfrac{12\times 2}{15\times 2}=\dfrac{24}{30}}$
Thus, ${\dfrac{4}{5}}$ and ${\dfrac{24}{30}}$ are two equivalent fractions of ${\dfrac{12}{15}}$