The derivative of hyperbolic functions represents the rate of change of the hyperbolic functions involving the combination of ex and e-x with respect to the variable.
It is used to describe the shape of electrical wires or cables hanging freely between two poles, as well as to model satellite rings and planetary formations.
Hyperbolic Sine (sinh)
As we know, the hyperbolic sine function, sinh x, is written as:
${\sinh x}$ = ${\dfrac{e^{x}-e^{-x}}{2}}$ …..(i)
Now, differentiating both sides of (i) with respect to x,
${\dfrac{d}{dx}\left( \sinh x\right) =\dfrac{d}{dx}\left( \dfrac{e^{x}-e^{-x}}{2}\right)}$
⇒ ${\dfrac{d}{dx}\left( \sinh x\right) =\dfrac{d}{dx}\left( \dfrac{e^{x}}{2}\right) -\dfrac{d}{dx}\left( \dfrac{e^{-x}}{2}\right)}$ …..(ii)
Since ${\dfrac{d}{dx}\left( e^{x}\right) =e^{x}}$ and ${\dfrac{d}{dx}\left( e^{-x}\right) =-e^{-x}}$ …..(iii)
Now, using (iii) in (ii), we get
⇒ ${\dfrac{d}{dx}\left( \sinh x\right) =\dfrac{1}{2}\left( e^{x}\right) -\dfrac{1}{2}\left( -e^{-x}\right)}$
⇒ ${\dfrac{d}{dx}\left( \sinh x\right) =\dfrac{e^{x}+e^{-x}}{2}}$
⇒ ${\dfrac{d}{dx}\left( \sinh x\right) =\cosh x}$
Hyperbolic Cosine (cosh)
As we know, the hyperbolic cosine function, cosh x, is written as:
${\cosh x}$ = ${\dfrac{e^{x}+e^{-x}}{2}}$ …..(i)
Differentiating both sides of (i) with respect to x,
${\dfrac{d}{dx}\left( \cosh x\right) =\dfrac{d}{dx}\left( \dfrac{e^{x}+e^{-x}}{2}\right)}$
⇒ ${\dfrac{d}{dx}\left( \cosh x\right) =\dfrac{d}{dx}\left( \dfrac{e^{x}}{2}\right) +\dfrac{d}{dx}\left( \dfrac{e^{-x}}{2}\right)}$ …..(ii)
Since ${\dfrac{d}{dx}\left( e^{x}\right) =e^{x}}$ and ${\dfrac{d}{dx}\left( e^{-x}\right) =-e^{-x}}$ …..(iii)
Now, using (iii) in (ii), we get
⇒ ${\dfrac{d}{dx}\left( \cosh x\right) =\dfrac{1}{2}\left( e^{x}\right) +\dfrac{1}{2}\left( -e^{-x}\right)}$
⇒ ${\dfrac{d}{dx}\left( \cosh x\right) =\dfrac{e^{x}-e^{-x}}{2}}$
⇒ ${\dfrac{d}{dx}\left( \cosh x\right) =\sinh x}$
Hyperbolic Tangent (tanh)
We can now obtain the derivative formula for hyperbolic tangent functions using the hyperbolic sine and cosine functions:
${\tanh x}$ = ${\dfrac{\sinh x}{\cosh x}}$ …..(i)
Differentiating both sides of (i) with respect to x,
${\dfrac{d}{dx}\left( \tanh x\right) =\dfrac{d}{dx}\left( \dfrac{\sinh x}{\cosh x}\right)}$
= ${\dfrac{\dfrac{d}{dx}\left( \sinh x\right) \cosh x-\sinh x\dfrac{d}{dx}\left( \cosh x\right) }{\cosh ^{2}x}}$ …..(ii)
As we know, the derivative of sinh x is cosh x, and the derivative of cosh x is sinh x.
Thus, from (ii), we get
${\dfrac{d}{dx}\left( \tanh x\right) =\dfrac{\cosh ^{2}x-\sinh ^{2}x}{\cosh ^{2}x}}$
= ${\dfrac{1}{\cosh ^{2}x}}$ (since, cosh2 x – sinh2 x = 1)
= ${sech ^{2}x}$ (since, ${\dfrac{1}{\cosh x}}$ = ${sech \ x}$)
Thus, ${\dfrac{d}{dx}\left( \tanh x\right) =sech ^{2}x}$
In addition to the basic function, the derivatives of three other hyperbolic functions are as follows:
Hyperbolic Cosecant (cosech)
As we know, cosech x is the reciprocal function of sinh x; thus
${cosech \ x}$ = ${\dfrac{1}{\sinh x}}$ …..(i)
Now, differentiating both sides of (i) with respect to x,
${\dfrac{d}{dx}\left( cosech \ x\right) =\dfrac{d}{dx}\left( \dfrac{1}{\sinh x}\right)}$
= ${\dfrac{\dfrac{d}{dx}\left( 1\right) \sinh x-\left( 1\right) \dfrac{d}{dx}\left( \sinh x\right) }{\sinh ^{2}x}}$
= ${-\dfrac{1}{\sinh x}\cdot \dfrac{coshx}{\sinh x}}$
= ${-cosech \ x\cdot \coth x}$
Thus, ${\dfrac{d}{dx}\left( cosech \ x\right) =-cosech \ x\cdot \coth x}$
Hyperbolic Secant (sech)
As we know, sech x is the reciprocal function of cosh x; thus
${sech \ x}$ = ${\dfrac{1}{\cosh x}}$ …..(i)
Now, differentiating both sides of (i) with respect to x,
${\dfrac{d}{dx}\left( sech \ x\right) =\dfrac{d}{dx}\left( \dfrac{1}{\cosh x}\right)}$
= ${\dfrac{\dfrac{d}{dx}\left( 1\right) \cosh x-\left( 1\right) \dfrac{d}{dx}\left( \cosh x\right) }{\cosh ^{2}x}}$
= ${-\dfrac{\sinh x}{\cosh ^{2}x}}$ (since, ${\dfrac{d}{dx}\left( 1\right) =0}$)
= ${-\dfrac{1}{\cosh x}\cdot \dfrac{sinhx}{\cosh x}}$
= ${-sech \ x\cdot \tanh x}$
Thus, ${\dfrac{d}{dx}\left( sech \ x\right) =-sech \ x\cdot \tanh x}$
Hyperbolic Cotangent (coth)
As we know, coth x is the reciprocal function of tanh x. Similarly, coth x can be expressed as:
${\coth x}$ = ${\dfrac{\cosh x}{\sinh x}}$ …..(i)
Differentiating both sides of (i) with respect to x,
${\dfrac{d}{dx}\left( \coth x\right) =\dfrac{d}{dx}\left( \dfrac{\cosh x}{\sinh x}\right)}$
= ${\dfrac{\dfrac{d}{dx}\left( \cosh x\right) \sinh x-\cosh x\dfrac{d}{dx}\left( \sinh x\right) }{\sinh ^{2}x}}$
= ${\dfrac{d}{dx}\left( \tanh x\right) =\dfrac{\sinh ^{2}x-\cosh ^{2}x}{\sinh ^{2}x}}$ (since, ${\dfrac{d}{dx}\left( \sinh x\right) =\cosh x}$ and ${\dfrac{d}{dx}\left( \cosh x\right) =\sinh x}$)
= ${-\dfrac{\left( \cosh ^{2}x-\sinh ^{2}x\right) }{\sinh ^{2}x}}$
= ${-\dfrac{1}{\sinh ^{2}x}}$ (since, cosh2 x – sinh2 x = 1)
= ${-cosech ^{2}x}$ (since, ${\dfrac{1}{\sinh x}}$ = ${cosech \ x}$)
Thus, ${\dfrac{d}{dx}\left( \coth x\right) =-cosech ^{2}x}$
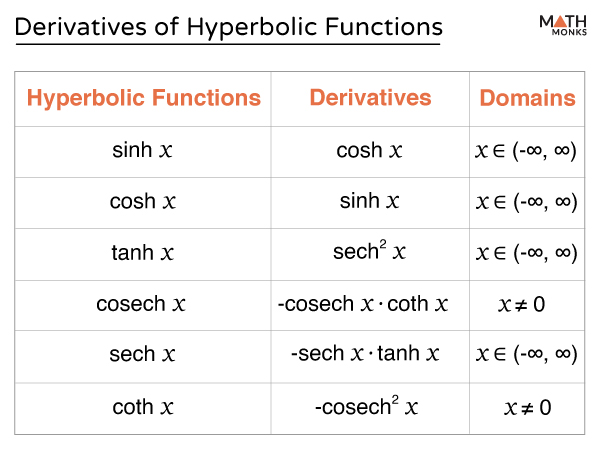
Here is a summary of all the derivatives of the hyperbolic functions with their domains:
Derivative of Inverse Hyperbolic Functions
Similar to finding the derivative of the hyperbolic functions, we can find the derivatives of the inverse hyperbolic functions using the inverse function theorem.
Inverse Hyperbolic Sine (arcsinh)
If x = sinh y …..(i)
Then, from the inverse function theorem, we get
y = arcsinh x
Now, to derive the inverse hyperbolic sine function (arcsinh), we need to use the derivative of the sinh function:
${\dfrac{d}{dx}\left( \sinh x\right) =\cosh x}$
Differentiating both sides of (i) with respect to x,
⇒ ${\dfrac{d}{dx}\left( x\right) =\dfrac{d}{dx}\left( \sinh y\right)}$
⇒ ${1=\cosh y\cdot \dfrac{dy}{dx}}$
⇒ ${\dfrac{dy}{dx}=\dfrac{1}{\cosh y}}$ …..(ii)
Using hyperbolic trigonometric identity, we have
cosh2 x – sinh2 x = 1 ⇒ cosh2 x = 1 + sinh2 x …..(iii)
Substituting the value (iii) in (ii), we get
⇒ ${\dfrac{dy}{dx}=\dfrac{1}{\sqrt{1+\sinh ^{2}y}}}$
⇒ ${\dfrac{dy}{dx}=\dfrac{1}{\sqrt{1+x^{2}}}}$
Thus, ${\dfrac{d}{dx}\left( arcsinh \ x\right) =\dfrac{1}{\sqrt{1+x^{2}}}}$
Inverse Hyperbolic Cosine (arccosh)
If x = cosh y …..(i)
Then, from the inverse function theorem, we get
y = arccosh x
Now, to derive the inverse hyperbolic cosine function (arccosh), we need to use the derivative of the cosh function:
${\dfrac{d}{dx}\left( \cosh x\right) =\sinh x}$
Now, differentiating both sides of (i) with respect to x,
⇒ ${\dfrac{d}{dx}\left( x\right) =\dfrac{d}{dx}\left( \cosh y\right)}$
⇒ ${1=\sinh y\cdot \dfrac{dy}{dx}}$
⇒ ${\dfrac{dy}{dx}=\dfrac{1}{\sinh y}}$ …..(ii)
Using hyperbolic trigonometric identity, we have
cosh2 x – sinh2 x = 1 ⇒ sinh2 x = cosh2 x – 1 …..(iii)
Substituting the value (iii) in (ii), we get
⇒ ${\dfrac{dy}{dx}=\dfrac{1}{\sqrt{\cosh ^{2}y-1}}}$
⇒ ${\dfrac{dy}{dx}=\dfrac{1}{\sqrt{x^{2}-1}}}$
Thus, ${\dfrac{d}{dx}\left( arccosh \ x\right) =\dfrac{1}{\sqrt{x^{2}-1}}}$, here x > 1
Inverse Hyperbolic Tangent (arctanh)
If x = tanh y …..(i)
Then, from the inverse function theorem, we get
y = arctanh x
Now, to derive the inverse hyperbolic tangent function (arctanh), we need to use the derivative of the tanh function:
${\dfrac{d}{dx}\left( \tanh x\right) =sech ^{2}x}$
Differentiating both sides of (i) with respect to x,
⇒ ${\dfrac{d}{dx}\left( x\right) =\dfrac{d}{dx}\left( \tanh y\right)}$
⇒ ${1=sech ^{2}y\cdot \dfrac{dy}{dx}}$
⇒ ${\dfrac{dy}{dx}=\dfrac{1}{sech ^{2}y}}$ …..(ii)
As we know the hyperbolic identity tanh2 x + sech2 x = 1 ⇒ sech2 x = 1 – tanh2 x …..(iii)
Using (iii) in (ii), we get
⇒ ${\dfrac{dy}{dx}=\dfrac{1}{1-\tanh ^{2}y}}$
⇒ ${\dfrac{dy}{dx}=\dfrac{1}{1-x^{2}}}$
Thus, ${\dfrac{d}{dx}\left( arctanh \ x\right) =\dfrac{1}{1-x^{2}}}$, here |x| < 1
Inverse Hyperbolic Cosecant (arccosech)
Now, to find the derivative of arccosech x, we will use the derivative of the hyperbolic cosecant function (cosech).
If y = arccosech x
⇒ x = cosech y …..(i)
Differentiating both sides of (i) with respect to x,
⇒ ${\dfrac{d}{dx}\left( x\right) =\dfrac{d}{dx}\left( cosech \ y\right)}$
Since, ${\dfrac{d}{dx}\left( cosech \ x\right) =-cosech \ x\cdot \coth x}$
⇒ ${1=-cosech \ y\cdot \coth y\cdot \dfrac{dy}{dx}}$
⇒ ${\dfrac{dy}{dx}=-\dfrac{1}{cosech \ y\cdot \coth y}}$ …..(ii)
As we know the hyperbolic identity coth2 x – cosech2 x = 1 ⇒ coth2 x = 1 + cosech2 x …..(iii)
Using (iii) in (ii), we get
⇒ ${\dfrac{dy}{dx}=-\dfrac{1}{cosech \ y\cdot \sqrt{1+cosech ^{2}y }}}$
⇒ ${\dfrac{dy}{dx}=-\dfrac{1}{\left| x\right| \sqrt{1-x^{2} }}}$
Thus, ${\dfrac{d}{dx}\left( arccosech \ x\right) =-\dfrac{1}{\left| x\right| \sqrt{1-x^{2} }}}$, here x ≠ 0
Inverse Hyperbolic Secant (arcsech)
Similarly, the derivative of arcsech x can be determined using the derivative of the hyperbolic secant function (sech).
If y = arcsech x
⇒ x = sech y …..(i)
Differentiating both sides of (i) with respect to x,
⇒ ${\dfrac{d}{dx}\left( x\right) =\dfrac{d}{dx}\left( sech \ y\right)}$
Since, ${\dfrac{d}{dx}\left( sech \ x\right) =-sech \ x\cdot \tanh x}$
⇒ ${1=-sech \ y\cdot \tanh y\cdot \dfrac{dy}{dx}}$
⇒ ${\dfrac{dy}{dx}=-\dfrac{1}{sech \ y\cdot \tanh y}}$ …..(ii)
As we know the hyperbolic identity tanh2 x + sech2 x = 1 ⇒ tanh2 x = 1 – sech2 x …..(iii)
Using (iii) in (ii), we get
⇒ ${\dfrac{dy}{dx}=-\dfrac{1}{sech \ y\cdot \sqrt{1-sech ^{2}y }}}$
⇒ ${\dfrac{dy}{dx}=-\dfrac{1}{x\sqrt{1-x^{2} }}}$
Thus, ${\dfrac{d}{dx}\left( arcsech \ x\right) =-\dfrac{1}{x\sqrt{1-x^{2} }}}$, here 0 < x < 1
Inverse Hyperbolic Cotangent (arccoth)
Now, we will derive the derivative of arccoth x using the derivative of the hyperbolic cotangent function (coth).
If y = arccoth x
⇒ x = coth y …..(i)
Differentiating both sides of (i) with respect to x,
⇒ ${\dfrac{d}{dx}\left( x\right) =\dfrac{d}{dx}\left( \coth y\right)}$
Since, ${\dfrac{d}{dx}\left( \coth x\right) =-cosech ^{2}x}$
⇒ ${1=-cosech ^{2}y\cdot \dfrac{dy}{dx}}$
⇒ ${\dfrac{dy}{dx}=\dfrac{1}{-cosech ^{2}y}}$ …..(ii)
As we know the hyperbolic identity coth2 x – cosech2 x = 1 ⇒ -cosech2 x = 1 – coth2 x …..(iii)
Using (iii) in (ii), we get
⇒ ${\dfrac{dy}{dx}=\dfrac{1}{1-\coth ^{2}y}}$
⇒ ${\dfrac{dy}{dx}=\dfrac{1}{1-x^{2}}}$
Thus, ${\dfrac{d}{dx}\left( arccoth \ x\right) =\dfrac{1}{1-x^{2}}}$, here |x| > 1
Here is a summary of derivatives of all the inverse hyperbolic functions with their domains:
Solved Examples
![]() Find the derivatives of the following hyperbolic function:
Find the derivatives of the following hyperbolic function:
ln(tanh (x3 + 2))
Solution:
![]()
Given, f(x) = ln(tanh (x3 + 2))
Now, differentiating with respect to x,
f’(x) = ${\dfrac{1}{\tanh \left( x^{3}+2\right) }\times \left( sech ^{2}\left( x^{3}+2\right) \right) \times \left( 3x^{2}\right)}$
= ${\dfrac{3x^{2}sech ^{2}\left( x^{3}+2\right) }{\tanh \left( x^{3}+2\right) }}$
![]() Find the derivatives of the inverse hyperbolic function:
Find the derivatives of the inverse hyperbolic function:
cosh-1(e3x)
Solution:
![]()
Given, f(x) = cosh-1(e3x)
Now, differentiating with respect to x,
f’(x) = ${\dfrac{1}{\sqrt{\left( e^{3r}\right) ^{2}-1}}\times \left( 3e^{3r}\right)}$
= ${\dfrac{3e^{3x}}{\sqrt{e^{6x}-1}}}$