Laguerre polynomials are a sequence of orthogonal polynomials, denoted by Ln(x), that arise as solutions to the Laguerre differential equation:
${x\dfrac{d^{2}y}{dx^{2}}+\left( 1-x\right) \dfrac{dy}{dx}+ny=0}$
Here,
- n is a non-negative integer (i.e., n = 0, 1, 2, 3, …)
Formula
The formula of Laguerre polynomials Ln(x) is written as:
${L_{n}\left( x\right) =\sum ^{n}_{k=0}\dfrac{\left( -1\right) ^{k}}{k!}\begin{pmatrix} n \\ k \end{pmatrix}x^{k}}$
Here,
- ${\begin{pmatrix} n \\ k \end{pmatrix}}$ represents the binomial coefficient
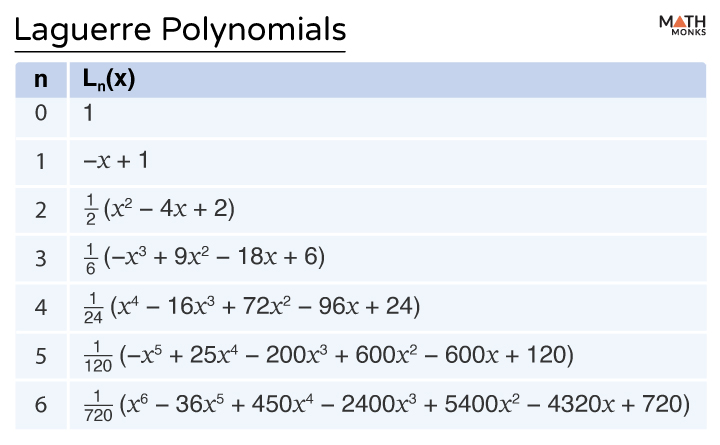
A few examples of Laguerre polynomials are listed:
Properties
Orthogonality
Laguerre polynomials are orthogonal over [0, ∞) with respect to the weight function e-x:
${\int ^{\infty }_{0}e^{-x}L_{m}\left( x\right) L_{n}\left( x\right) dx=0}$ for m ≠ n
Rodrigues’ Formula
These polynomials can be explicitly defined using Rodrigues’ formula:
${L_{n}\left( x\right) =\dfrac{e^{x}}{n!}\dfrac{d^{n}}{dx^{n}}\left( e^{-x}x^{n}\right)}$
Recurrence Relations
Laguerre polynomials satisfy the following recurrence relations:
(n + 1)Ln + 1(x) = (2n + 1 – x)Ln(x) – nLn – 1(x)
${x\dfrac{d}{dx}L_{n}\left( x\right) =nL_{n}\left( x\right) -nL_{n-1}\left( x\right)}$
Generating Function
The generating function for Laguerre polynomials is used to derive the polynomials:
${\sum ^{\infty }_{n=0}L_{n}\left( x\right) t^{n}=\dfrac{e^{-xt}}{1-t},\left| t\right| <1}$
Differentiation
The derivative of the nth-order Laguerre polynomial is:
${\dfrac{d}{dx}L_{n}\left( x\right) =-L_{n-1}\left( x\right)}$
Relation to Associated Laguerre Polynomials
The associated Laguerre polynomials, Ln(α)(x), generalize the standard Laguerre polynomials and are defined as:
${L_{n}^{\left( \alpha \right) }\left( x\right) =\dfrac{e^{x}x^{-\alpha }}{n!}\dfrac{d^{n}}{dx^{n}}\left( e^{-x}x^{n+\alpha }\right)}$
They are orthogonal with respect to the weight function xαe-x over the interval [0, ∞):
${\int ^{\infty }_{0}e^{-x}x^{\alpha }L_{n}^{\left( \alpha \right) }\left( x\right) L_{m}^{\left( \alpha \right) }\left( x\right) dx=\dfrac{\left( n+\alpha \right) !}{n!}\delta _{mn}}$
Here,
- δmn is the Kronecker delta
Solved Examples
![]()
Find the second Laguerre polynomial L2(x)
Solution:
![]()
As we know, ${L_{n}\left( x\right) =\sum ^{n}_{k=0}\dfrac{\left( -1\right) ^{k}}{k!}\begin{pmatrix} n \\ k \end{pmatrix}x^{k}}$
For n = 2,
L2(x) = ${\dfrac{\left( -1\right) ^{0}}{0!}\begin{pmatrix} 2 \\ 0 \end{pmatrix}x^{0}+\dfrac{\left( -1\right) ^{1}}{1!}\begin{pmatrix} 2 \\ 1 \end{pmatrix}x^{1}+\dfrac{\left( -1\right) ^{2}}{2!}\begin{pmatrix} 2 \\ 2 \end{pmatrix}x^{2}}$
⇒ L2(x) = ${1-2x+\dfrac{x^{2}}{2}}$
⇒ L2(x) = ${\dfrac{1}{2}\left( x^{2}-4x+2\right)}$
Thus, L2(x) = ${\dfrac{1}{2}\left( x^{2}-4x+2\right)}$
![]()
Find the third Laguerre polynomial L3(x)
Solution:
![]()
As we know, ${L_{n}\left( x\right) =\sum ^{n}_{k=0}\dfrac{\left( -1\right) ^{k}}{k!}\begin{pmatrix} n \\ k \end{pmatrix}x^{k}}$
For n = 3,
L3(x) = ${\dfrac{\left( -1\right) ^{0}}{0!}\begin{pmatrix} 3 \\ 0 \end{pmatrix}x^{0}+\dfrac{\left( -1\right) ^{1}}{1!}\begin{pmatrix} 3 \\ 1 \end{pmatrix}x^{1}+\dfrac{\left( -1\right) ^{2}}{2!}\begin{pmatrix} 3 \\ 2 \end{pmatrix}x^{2}+\dfrac{\left( -1\right) ^{3}}{3!}\begin{pmatrix} 3 \\ 3 \end{pmatrix}x^{3}}$
⇒ L3(x) = ${1-3x+\dfrac{3x^{2}}{2}-\dfrac{x^{3}}{6}}$
⇒ L3(x) = ${\dfrac{1}{6}\left( -x^{3}+9x^{2}-18x+6\right)}$
Thus, L3(x) = ${\dfrac{1}{6}\left( -x^{3}+9x^{2}-18x+6\right)}$
