This article will help us learn how to prove something is a parallelogram. More precisely, how to prove a quadrilateral is a parallelogram.
There are 5 basic ways to prove a quadrilateral is a parallelogram. They are as follows:
- Proving opposite sides are congruent
- Proving opposite sides are parallel
- Proving the quadrilateral’s diagonals bisect each other
- Proving opposite angles are congruent
- Proving consecutive angles are supplementary (adding to 180°)
Let us now prove the above statements one by one.
1) Proving Opposite Sides are Congruent
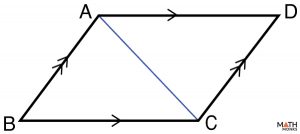
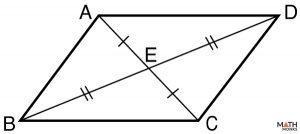
Prove that opposite sides of a parallelogram are congruent
To prove: AB ≅ CD, AD ≅ BC
Proof:
Given: AB ∥ CD, AD ∥ BC
Draw in a diagonal AC
| Statement | Reason |
|---|
| ∠ACB ≅ ∠CAD | Alternate interior angles |
| ∠BAC ≅ ∠ACD | Alternate interior angles |
| AC ≅ AC | Common side (identity) |
| ΔADC ≅ ΔABC | Angle-Angle-Side (AAS) postulate |
| AD ≅ BC AB ≅ CD | Corresponding Parts of Congruent Triangles are Congruent (CPCTC) Hence Proved |
2) Proving Opposite Sides are Parallel
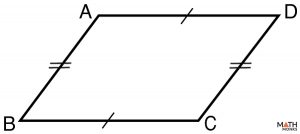
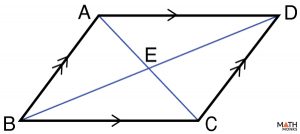
Prove that opposite sides of a parallelogram are parallel
To prove: AB ∥ CD, AD ∥ BC
Proof:
Given: AB ≅ CD, AD ≅ BC
Draw in a diagonal AC
| Statement | Reason |
|---|
| AC ≅ AC | Common side (identity) |
| ΔADC ≅ ΔABC | SSS postulate |
| ∠ACB ≅ ∠CAD | CPCTC |
| ∠BAC ≅ ∠ACD | CPCTC |
| AB ∥ CD | Alternate interior angles Hence Proved |
| AD ∥ BC | Alternate interior angles Hence Proved |
3) Proving Diagonals Bisect Each Other
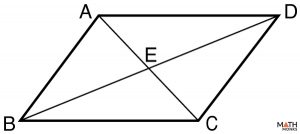
Prove that the diagonals of a parallelogram bisect each other.
This is an “if and only if” proof, so there are two things to prove.
1. To prove: AE ≅ EC, BE ≅ ED
Proof:
Given: ABCD is a parallelogram
| Statement | Reason |
|---|
| AD ∥ BC | Definition of parallelogram |
| ∠DAE ≅ ∠BCE | Alternate interior angles |
| ADE ≅ CBE | Alternate interior angles |
| AD ≅ BC | Opposite sides of parallelogram |
| ΔADC ≅ ΔABC | AAS postulate |
| AE ≅ EC | CPCTC Hence Proved |
| BE ≅ ED | CPCTC Hence Proved |
And the converse
2. To prove: ABCD is a parallelogram
Proof:
Given: AE ≅ EC, BE ≅ ED
| Statement | Reason |
|---|
| ∠AED ≅ ∠BEC | Vertical angles |
| ∠AEB ≅ ∠CED | Vertical angles |
| ΔADC ≅ ΔABC | AAS |
| AD ≅ BC | CPCTC |
| AB ≅ CD | CPCTC |
| ABCD is a parallelogram | Theorem 2 Hence Proved |
4) Proving Opposite Angles are Congruent
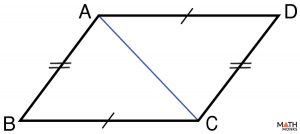
Prove that the opposite angles of a parallelogram are congruent.
To prove: ∠ADC ≅ ∠ABC, ∠BAD ≅ ∠BCD
Proof:
Given: AB ∥ CD, AD ∥ BC
Draw in a diagonal AC
| Statement | Reason |
|---|
| ∠ACB ≅ ∠CAD | Alternate interior angles |
| ∠ADB ≅ ∠CBD | Alternate interior angles |
| ∠AED ≅ ∠BEC | Vertical angles |
| ∠AEB ≅ ∠CED | Vertical angles |
| ΔADC ≅ ΔABC | AAS postulate |
| ΔBAD ≅ ΔBCD | AAS postulate |
| ∠ADC ≅ ∠ABC | CPCTC postulate Hence Proved |
| ∠BAD ≅ ∠BCD | CPCTC postulate Hence Proved |
5) Proving Consecutive Angles are Supplementary
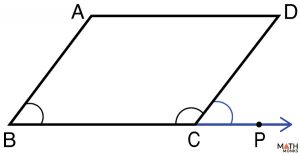
Prove that the consecutive angles of a parallelogram are supplementary (add up to 180°).
To prove: ∠ABC + ∠BCD = 180°
Proof:
Given: AB ∥ CD, AD ∥ BC
Extend BC till P
| Statement | Reason |
|---|
| ∠ABC ≅ ∠DCP | Corresponding angle |
| ∠BCD + ∠DCP = 180° | Linear angle |
| ∠ABC + ∠BCD = 180° | ∠ABC ≅ ∠DCP Hence Proved |