Gamma Function

The Gamma Function is a function that extends the concept of factorials beyond whole numbers. As we know, the formula of the factorials is: 

n! = n × (n – 1) × (n – 2) × ⋯ × 2 × 1

It works well for whole numbers like 1, 2, and 3. However, to find ${\left( \dfrac{1}{2}\right) !}$ or (-3.5)!, we need the Gamma Function. The Gamma Function is represented by the symbol Γ(n) (read as ‘gamma of n’).

Thus, it is a generalized version of the factorial function that works for all real and complex numbers except non-positive integers.

Mathematically, it is defined as:

${\Gamma \left( z\right) =\int ^{\infty }_{0}x^{z-1}e^{-x}dx}$

The Gamma Function is used in probability and statistics like gamma and chi-square distributions, as well as in complex analysis, including Bessel and Legendre functions.

Properties

Recurrence Relation 

Just like n! = n × (n-1)!, the gamma function satisfies the recurrence relation:

Γ(z+1) = z Γ(z), which allows us to calculate values step by step. 

For example,

Γ(3.5) = 2.5 Γ(2.5) = 2.5 × 1.5 Γ(1.5)

This makes calculations easier without directly integrating the function.

Value at Half-Integer Points

The Gamma function has a well-known value at half-integer points:

${\Gamma \left( \dfrac{1}{2}\right) =\sqrt{\pi }}$

This result is essential in probability and statistics, particularly in the normal distribution and beta function.

Reflection Formula

This formula helps find gamma function values for negative inputs:

${\Gamma \left( z\right) \Gamma \left( 1-z\right) =\dfrac{\pi }{\sin \left( \pi z\right) }}$

Behavior for Negative Values

The Gamma Function is not defined for negative integers (e.g., Γ(-1), Γ(-2), Γ(-3), …) because it has poles at these points (tends to infinity). However, for negative non-integer values, the function is well-defined and can be computed using the reflection formula.

This formula allows us to compute Gamma values for negative non-integers using their positive counterparts.

For example,

${\Gamma \left( -\dfrac{1}{2}\right) \Gamma \left( 1+\dfrac{1}{2}\right) =\dfrac{\pi }{\sin \left( \pi \left( -\dfrac{1}{2}\right) \right) }}$

⇒ ${\Gamma \left( -\dfrac{1}{2}\right) =\dfrac{\pi }{\sin \left( -\dfrac{\pi }{2}\right) \times \Gamma \left( \dfrac{3}{2}\right) }}$

⇒ ${\Gamma \left( -\dfrac{1}{2}\right) =\dfrac{\pi }{-1\times \dfrac{\sqrt{\pi }}{2}}}$

⇒ ${\Gamma \left( -\dfrac{1}{2}\right) =-\dfrac{2\sqrt{\pi }}{\sqrt{\pi }}}$

⇒ ${\Gamma \left( -\dfrac{1}{2}\right) =-2}$

Evaluating Gamma Function

Let us evaluate the value of Γ(5) using the recurrence relation:

As we know, Γ(n) = (n – 1)!

Here, Γ(5) = (5 – 1)! = 4! = 4 × 3 × 2 × 1 = 24

Thus, Γ(5) = 24

For Fractions

For fractional values, the gamma function formula becomes:

${\Gamma \left( \dfrac{1}{2}\right) =\sqrt{\pi }}$

By using the recurrence relation: ${\Gamma \left( z+1\right) =z\Gamma \left( z\right)}$

We can determine values for other fractions as well. 

For example,

${\Gamma \left( \dfrac{3}{2}\right) =\dfrac{1}{2}\Gamma \left( \dfrac{1}{2}\right) =\dfrac{\sqrt{\pi }}{2}}$

${\Gamma \left( \dfrac{5}{2}\right) =\dfrac{3}{2}\Gamma \left( \dfrac{3}{2}\right) =\dfrac{3}{2}\times \dfrac{\sqrt{\pi }}{2}=\dfrac{3\sqrt{\pi }}{4}}$

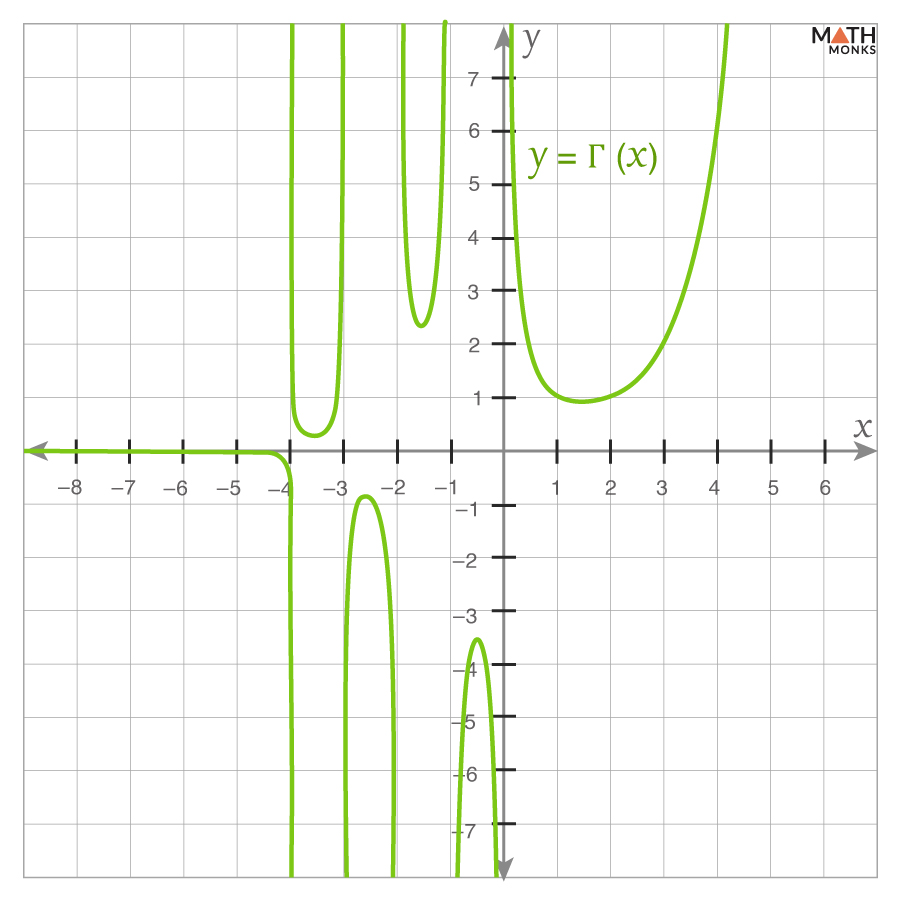
By Graphing

As we know, the Gamma Function extends the concept of factorials to non-integer and complex numbers. For positive integers, it follows the familiar pattern Γ(n) = (n – 1)!, but for non-integer values, it smoothly interpolates between these points.

However, at negative integers, it is undefined and diverges to infinity.

The graph below visually represents these key properties:

• For positive integers, Γ(x) follows factorial values.
• For fractional values, Γ(x) smoothly varies without abrupt jumps.
• At negative integers, vertical asymptotes (green dashed lines) indicate poles where Γ(x) is undefined.

This visualization highlights how the Gamma Function generalizes factorials while also showing its limitations at negative integers.

Note: The Gamma Function also applies to complex numbers, provided that their real part is greater than zero.

Solved Examples