- Shapes
- Rectangle
- Square
- Circle
- Triangle
- Rhombus
- Squircle
- Oval
- Hexagon
- Pentagon
- Trapezoid
- Kite
- Parallelogram
- Quadrilateral
- Polygon
- Nonagon
- Heptagon
- Decagon
- Octagon
- Ellipse
- Parallelepiped
- Tetrahedron
- Cylinder
- Prism
- Sphere
- Pyramid
- Frustum
- Polyhedron
- Dodecagon
- Dodecahedron
- Octahedron
- Torus
- Cube
- Cone
- Hyperbola
- Rectangular Prism
- Fibonacci Sequence
- Golden Ratio
- Parabola
- Worksheets
- Calculators
- Angle
- Arithmetic
- Whole Numbers
- Rational Numbers
- Place Value
- Irrational Numbers
- Natural Numbers
- Binary Operation
- Numerator and Denominator
- Decimal
- Order of Operations (PEMDAS)
- Scientific Notation
- Symmetry
- Fractions
- Triangular Number
- Complex Number
- Binary Number System
- Logarithm
- Binomial Theorem
- Quartic Function
- Mathematical Induction
- Group Theory
- Modular Arithmetic
- Euler’s Number
- Inequalities
- Sets
- De Morgan’s Laws
- Transcendental Numbers
- About Us
Search


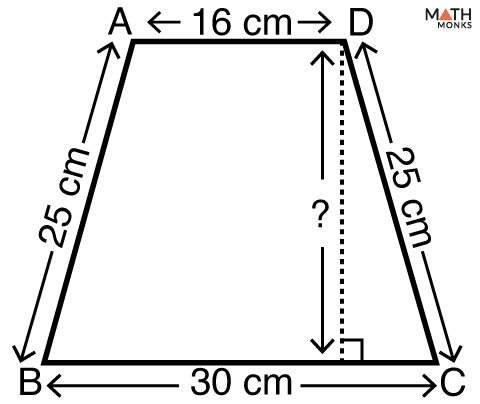 Find the height of an isosceles trapezoid with bases 30 cm and 16 cm, and legs 25 cm each.
Find the height of an isosceles trapezoid with bases 30 cm and 16 cm, and legs 25 cm each.