- Shapes
- Rectangle
- Square
- Circle
- Triangle
- Rhombus
- Squircle
- Oval
- Hexagon
- Pentagon
- Trapezoid
- Kite
- Parallelogram
- Quadrilateral
- Polygon
- Nonagon
- Heptagon
- Decagon
- Octagon
- Ellipse
- Parallelepiped
- Tetrahedron
- Cylinder
- Prism
- Sphere
- Pyramid
- Frustum
- Polyhedron
- Dodecagon
- Dodecahedron
- Octahedron
- Torus
- Cube
- Cone
- Hyperbola
- Rectangular Prism
- Fibonacci Sequence
- Golden Ratio
- Parabola
- Worksheets
- Calculators
- Angle
- Arithmetic
- Whole Numbers
- Rational Numbers
- Place Value
- Irrational Numbers
- Natural Numbers
- Binary Operation
- Numerator and Denominator
- Decimal
- Order of Operations (PEMDAS)
- Scientific Notation
- Symmetry
- Fractions
- Triangular Number
- Complex Number
- Binary Number System
- Logarithm
- Binomial Theorem
- Quartic Function
- Mathematical Induction
- Group Theory
- Modular Arithmetic
- Euler’s Number
- Inequalities
- Sets
- De Morgan’s Laws
- Transcendental Numbers
- About Us
Search

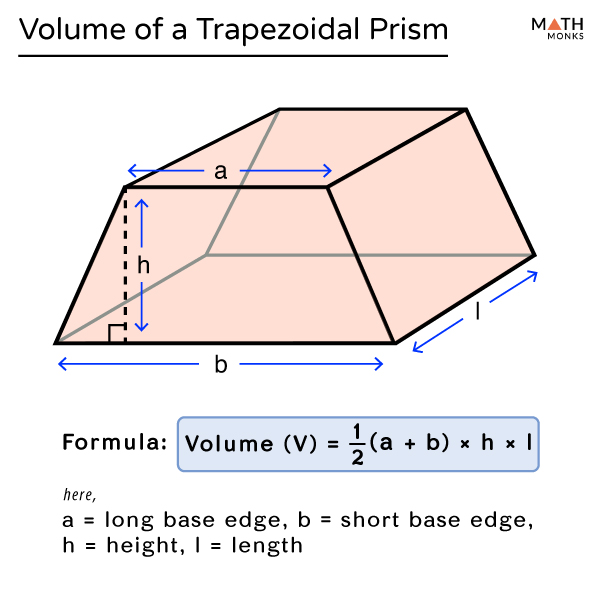
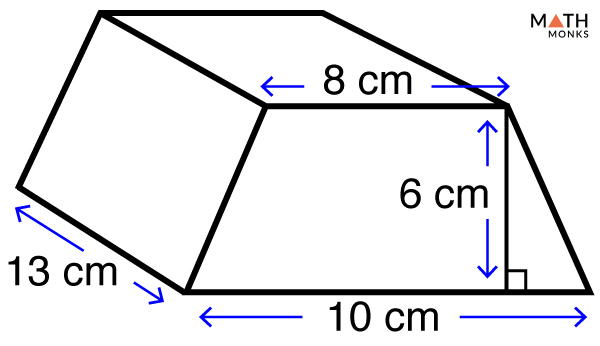 Find the volume of a trapezoidal prism given in the figure.
Find the volume of a trapezoidal prism given in the figure.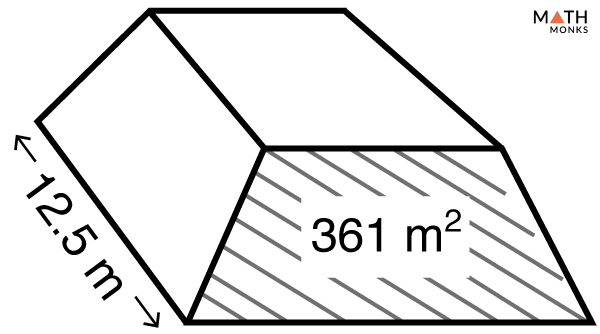 Find the volume of a trapezoidal prism given in the figure, whose base area is 361 m2 and length is 12.5 m.
Find the volume of a trapezoidal prism given in the figure, whose base area is 361 m2 and length is 12.5 m.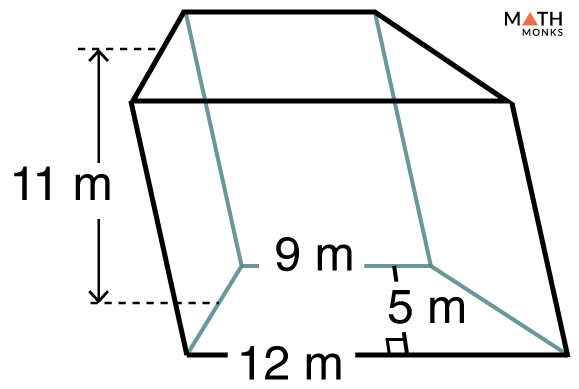 Find the volume of an oblique trapezoidal prism given in the figure.
Find the volume of an oblique trapezoidal prism given in the figure.
This is an interesting and informative post on the volume of a trapezoidal prism. It provides a clear definition, formula, and examples to help readers understand this concept better. Thanks for sharing!